ベルマン フォード アルゴリズムは、単一ソースの最短パス アルゴリズムです。このアルゴリズムは、重み付きグラフの単一の頂点から他のすべての頂点までの最短距離を見つけるために使用されます。ダイクストラ アルゴリズムなど、最短経路を見つけるために使用される他のさまざまなアルゴリズムがあります。重み付けされたグラフに負の重み値が含まれている場合、ダイクストラ アルゴリズムは正しい答えを生成するかどうかを確認しません。ダイクストラ アルゴリズムとは対照的に、ベルマン フォード アルゴリズムは、重み付けされたグラフに負の重み値が含まれている場合でも、正しい答えを保証します。
このアルゴリズムのルール
We will go on relaxing all the edges (n - 1) times where, n = number of vertices
以下のグラフを考えてみましょう。
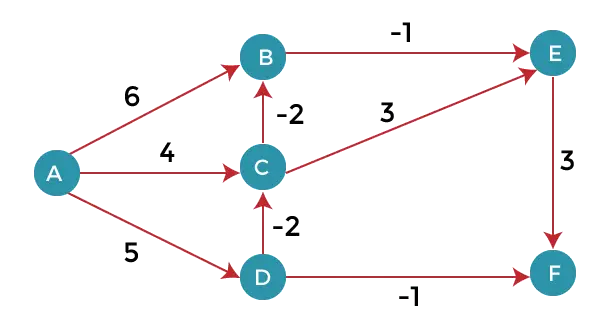
上のグラフでわかるように、一部の重みが負になっています。上のグラフには 6 つの頂点が含まれているため、5 つの頂点までリラックスを続けます。ここでは、すべてのエッジを 5 回緩めます。ループは 5 回繰り返され、正しい答えが得られます。ループが 5 回以上繰り返された場合も、答えは同じになります。つまり、頂点間の距離に変化はありません。
リラックスとは次のことを意味します。
メガバイトとギガバイトの違いは何ですか
If (d(u) + c(u , v) <d(v)) d(v)="d(u)" + c(u , v) < pre> <p>To find the shortest path of the above graph, the first step is note down all the edges which are given below:</p> <p>(A, B), (A, C), (A, D), (B, E), (C, E), (D, C), (D, F), (E, F), (C, B)</p> <p>Let's consider the source vertex as 'A'; therefore, the distance value at vertex A is 0 and the distance value at all the other vertices as infinity shown as below:</p> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-2.webp" alt="Bellman-Ford Algorithm"> <p>Since the graph has six vertices so it will have five iterations.</p> <p> <strong>First iteration</strong> </p> <p>Consider the edge (A, B). Denote vertex 'A' as 'u' and vertex 'B' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 6</p> <p>Since (0 + 6) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 6 = 6</p> <p>Therefore, the distance of vertex B is 6.</p> <p>Consider the edge (A, C). Denote vertex 'A' as 'u' and vertex 'C' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 4</p> <p>Since (0 + 4) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 4 = 4</p> <p>Therefore, the distance of vertex C is 4.</p> <p>Consider the edge (A, D). Denote vertex 'A' as 'u' and vertex 'D' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 5</p> <p>Since (0 + 5) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 5 = 5</p> <p>Therefore, the distance of vertex D is 5.</p> <p>Consider the edge (B, E). Denote vertex 'B' as 'u' and vertex 'E' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 6</p> <p>d(v) = ∞</p> <p>c(u , v) = -1</p> <p>Since (6 - 1) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 6 - 1= 5</p> <p>Therefore, the distance of vertex E is 5.</p> <p>Consider the edge (C, E). Denote vertex 'C' as 'u' and vertex 'E' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 4</p> <p>d(v) = 5</p> <p>c(u , v) = 3</p> <p>Since (4 + 3) is greater than 5, so there will be no updation. The value at vertex E is 5.</p> <p>Consider the edge (D, C). Denote vertex 'D' as 'u' and vertex 'C' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 5</p> <p>d(v) = 4</p> <p>c(u , v) = -2</p> <p>Since (5 -2) is less than 4, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 5 - 2 = 3</p> <p>Therefore, the distance of vertex C is 3.</p> <p>Consider the edge (D, F). Denote vertex 'D' as 'u' and vertex 'F' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 5</p> <p>d(v) = ∞</p> <p>c(u , v) = -1</p> <p>Since (5 -1) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 5 - 1 = 4</p> <p>Therefore, the distance of vertex F is 4.</p> <p>Consider the edge (E, F). Denote vertex 'E' as 'u' and vertex 'F' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 5</p> <p>d(v) = ∞</p> <p>c(u , v) = 3</p> <p>Since (5 + 3) is greater than 4, so there would be no updation on the distance value of vertex F.</p> <p>Consider the edge (C, B). Denote vertex 'C' as 'u' and vertex 'B' as 'v'. Now use the relaxing formula:</p> <p>d(u) = 3</p> <p>d(v) = 6</p> <p>c(u , v) = -2</p> <p>Since (3 - 2) is less than 6, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 3 - 2 = 1</p> <p>Therefore, the distance of vertex B is 1.</p> <p>Now the first iteration is completed. We move to the second iteration.</p> <p> <strong>Second iteration:</strong> </p> <p>In the second iteration, we again check all the edges. The first edge is (A, B). Since (0 + 6) is greater than 1 so there would be no updation in the vertex B.</p> <p>The next edge is (A, C). Since (0 + 4) is greater than 3 so there would be no updation in the vertex C.</p> <p>The next edge is (A, D). Since (0 + 5) equals to 5 so there would be no updation in the vertex D.</p> <p>The next edge is (B, E). Since (1 - 1) equals to 0 which is less than 5 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(E) = d(B) +c(B , E)</p> <p>= 1 - 1 = 0</p> <p>The next edge is (C, E). Since (3 + 3) equals to 6 which is greater than 5 so there would be no updation in the vertex E.</p> <p>The next edge is (D, C). Since (5 - 2) equals to 3 so there would be no updation in the vertex C.</p> <p>The next edge is (D, F). Since (5 - 1) equals to 4 so there would be no updation in the vertex F.</p> <p>The next edge is (E, F). Since (5 + 3) equals to 8 which is greater than 4 so there would be no updation in the vertex F.</p> <p>The next edge is (C, B). Since (3 - 2) equals to 1` so there would be no updation in the vertex B.</p> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-3.webp" alt="Bellman-Ford Algorithm"> <p> <strong>Third iteration</strong> </p> <p>We will perform the same steps as we did in the previous iterations. We will observe that there will be no updation in the distance of vertices.</p> <pre> The following are the distances of vertices: A: 0 B: 1 C: 3 D: 5 E: 0 F: 3 </pre> <p> <strong>Time Complexity</strong> </p> <p>The time complexity of Bellman ford algorithm would be O(E|V| - 1).</p> <pre> function bellmanFord(G, S) for each vertex V in G distance[V] <- 0 infinite previous[v] <- null distance[s] for each vertex v in g edge (u,v) tempdistance distance[u] + edge_weight(u, v) if < distance[v] u distance[v} error: negative cycle exists return distance[], previous[] pre> <p> <strong>Drawbacks of Bellman ford algorithm</strong> </p> <ul> <li>The bellman ford algorithm does not produce a correct answer if the sum of the edges of a cycle is negative. Let's understand this property through an example. Consider the below graph. <br> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-4.webp" alt="Bellman-Ford Algorithm"></li> <li>In the above graph, we consider vertex 1 as the source vertex and provides 0 value to it. We provide infinity value to other vertices shown as below: <br> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-5.webp" alt="Bellman-Ford Algorithm"> <br>Edges can be written as: <br> (1, 3), (1, 2), (2, 4), (3, 2), (4, 3) </li> </ul> <p> <strong>First iteration</strong> </p> <p> <strong>Consider the edge (1, 3). Denote vertex '1' as 'u' and vertex '3' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 5</p> <p>Since (0 + 5) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 5 = 5</p> <p>Therefore, the distance of vertex 3 is 5.</p> <p> <strong>Consider the edge (1, 2). Denote vertex '1' as 'u' and vertex '2' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 4</p> <p>Since (0 + 4) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 4 = 4</p> <p>Therefore, the distance of vertex 2 is 4.</p> <p> <strong>Consider the edge (3, 2). Denote vertex '3' as 'u' and vertex '2' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 5</p> <p>d(v) = 4</p> <p>c(u , v) = 7</p> <p>Since (5 + 7) is greater than 4, so there would be no updation in the vertex 2.</p> <p> <strong>Consider the edge (2, 4). Denote vertex '2' as 'u' and vertex '4' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 4</p> <p>d(v) = ∞</p> <p>c(u , v) = 7</p> <p>Since (4 + 7) equals to 11 which is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 4 + 7 = 11</p> <p>Therefore, the distance of vertex 4 is 11.</p> <p> <strong>Consider the edge (4, 3). Denote vertex '4' as 'u' and vertex '3' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 11</p> <p>d(v) = 5</p> <p>c(u , v) = -15</p> <p>Since (11 - 15) equals to -4 which is less than 5, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 11 - 15 = -4</p> <p>Therefore, the distance of vertex 3 is -4.</p> <p>Now we move to the second iteration.</p> <p> <strong>Second iteration</strong> </p> <p>Now, again we will check all the edges. The first edge is (1, 3). Since (0 + 5) equals to 5 which is greater than -4 so there would be no updation in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) equals to 4 so there would be no updation in the vertex 2.</p> <p>The next edge is (3, 2). Since (-4 + 7) equals to 3 which is less than 4 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -4 + 7 = 3</p> <p>Therefore, the value at vertex 2 is 3.</p> <p>The next edge is (2, 4). Since ( 3+7) equals to 10 which is less than 11 so update</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(4) = d(2) +c(2, 4)</p> <p>= 3 + 7 = 10</p> <p>Therefore, the value at vertex 4 is 10.</p> <p>The next edge is (4, 3). Since (10 - 15) equals to -5 which is less than -4 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(3) = d(4) +c(4, 3)</p> <p>= 10 - 15 = -5</p> <p>Therefore, the value at vertex 3 is -5.</p> <p>Now we move to the third iteration.</p> <p> <strong>Third iteration</strong> </p> <p>Now again we will check all the edges. The first edge is (1, 3). Since (0 + 5) equals to 5 which is greater than -5 so there would be no updation in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) equals to 4 which is greater than 3 so there would be no updation in the vertex 2.</p> <p>The next edge is (3, 2). Since (-5 + 7) equals to 2 which is less than 3 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -5 + 7 = 2</p> <p>Therefore, the value at vertex 2 is 2.</p> <p>The next edge is (2, 4). Since (2 + 7) equals to 9 which is less than 10 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(4) = d(2) +c(2, 4)</p> <p>= 2 + 7 = 9</p> <p>Therefore, the value at vertex 4 is 9.</p> <p>The next edge is (4, 3). Since (9 - 15) equals to -6 which is less than -5 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(3) = d(4) +c(4, 3)</p> <p>= 9 - 15 = -6</p> <p>Therefore, the value at vertex 3 is -6.</p> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-6.webp" alt="Bellman-Ford Algorithm"> <p>Since the graph contains 4 vertices, so according to the bellman ford algorithm, there would be only 3 iterations. If we try to perform 4<sup>th</sup> iteration on the graph, the distance of the vertices from the given vertex should not change. If the distance varies, it means that the bellman ford algorithm is not providing the correct answer.</p> <p> <strong>4<sup>th</sup> iteration</strong> </p> <p>The first edge is (1, 3). Since (0 +5) equals to 5 which is greater than -6 so there would be no change in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) is greater than 2 so there would be no updation.</p> <p>The next edge is (3, 2). Since (-6 + 7) equals to 1 which is less than 3 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -6 + 7 = 1</p> <p>In this case, the value of the vertex is updated. So, we conclude that the bellman ford algorithm does not work when the graph contains the negative weight cycle.</p> <p>Therefore, the value at vertex 2 is 1.</p> <hr></-></pre></d(v))>
d(v) = 0 + 6 = 6
したがって、頂点 B の距離は 6 になります。
エッジ (A、C) を考えてみましょう。頂点 'A' を 'u' として、頂点 'C' を 'v' として示します。ここでリラックス公式を使用します。
d(u) = 0
d(v) = ∞
c(u , v) = 4
(0 + 4) は ∞ 未満なので、更新します
d(v) = d(u) + c(u , v)
d(v) = 0 + 4 = 4
したがって、頂点 C の距離は 4 になります。
エッジ (A、D) を考えてみましょう。頂点 'A' を 'u' として、頂点 'D' を 'v' として示します。ここでリラックス公式を使用します。
d(u) = 0
d(v) = ∞
c(u , v) = 5
(0 + 5) は ∞ 未満なので、更新します
d(v) = d(u) + c(u , v)
d(v) = 0 + 5 = 5
したがって、頂点 D の距離は 5 になります。
エッジ (B、E) を考えてみましょう。頂点 'B' を 'u' として、頂点 'E' を 'v' として示します。ここでリラックス公式を使用します。
d(u) = 6
d(v) = ∞
c(u , v) = -1
(6 - 1) は ∞ より小さいので、更新します。
d(v) = d(u) + c(u , v)
d(v) = 6 - 1= 5
したがって、頂点 E の距離は 5 です。
エッジ (C、E) を考えてみましょう。頂点 'C' を 'u' として、頂点 'E' を 'v' として示します。ここでリラックス公式を使用します。
d(u) = 4
d(v) = 5
c(u , v) = 3
(4 + 3) は 5 より大きいため、更新は行われません。頂点 E の値は 5 です。
エッジ (D、C) を考えてみましょう。頂点 'D' を 'u' として表し、頂点 'C' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 5
d(v) = 4
c(u , v) = -2
(5 -2) は 4 未満なので、更新します
d(v) = d(u) + c(u , v)
d(v) = 5 - 2 = 3
したがって、頂点 C の距離は 3 になります。
エッジ (D、F) を考えてみましょう。頂点 'D' を 'u' として表し、頂点 'F' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 5
d(v) = ∞
c(u , v) = -1
(5 -1) は ∞ より小さいので、更新します。
d(v) = d(u) + c(u , v)
d(v) = 5 - 1 = 4
したがって、頂点 F の距離は 4 になります。
エッジ (E、F) を考えてみましょう。頂点 'E' を 'u' として、頂点 'F' を 'v' として示します。ここでリラックス公式を使用します。
文字列.値
d(u) = 5
d(v) = ∞
c(u , v) = 3
(5 + 3) は 4 より大きいため、頂点 F の距離値は更新されません。
エッジ (C、B) を考えてみましょう。頂点 'C' を 'u' として、頂点 'B' を 'v' として示します。ここでリラックス公式を使用します。
d(u) = 3
d(v) = 6
c(u , v) = -2
(3 - 2) は 6 未満なので更新します
d(v) = d(u) + c(u , v)
d(v) = 3 - 2 = 1
したがって、頂点 B の距離は 1 になります。
これで最初の反復が完了しました。 2 番目の反復に進みます。
2 回目の反復:
2 回目の反復では、すべてのエッジを再度チェックします。最初のエッジは (A, B) です。 (0 + 6) は 1 より大きいため、頂点 B は更新されません。
次のエッジは (A, C) です。 (0 + 4) は 3 より大きいため、頂点 C は更新されません。
次のエッジは (A, D) です。 (0 + 5) は 5 に等しいため、頂点 D には更新はありません。
次のエッジは (B, E) です。 (1 - 1) は 5 未満の 0 に等しいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(E) = d(B) +c(B , E)
= 1 - 1 = 0
次のエッジは (C, E) です。 (3 + 3) は 5 より大きい 6 に等しいため、頂点 E は更新されません。
次のエッジは (D, C) です。 (5 - 2) は 3 に等しいため、頂点 C には更新はありません。
次のエッジは (D, F) です。 (5 - 1) は 4 に等しいため、頂点 F には更新はありません。
次のエッジは (E, F) です。 (5 + 3) は 4 より大きい 8 に等しいため、頂点 F は更新されません。
次のエッジは (C, B) です。 (3 - 2) は 1` に等しいため、頂点 B には更新はありません。

3回目の反復
前回の反復で行ったのと同じ手順を実行します。頂点の距離が更新されないことが観察されます。
The following are the distances of vertices: A: 0 B: 1 C: 3 D: 5 E: 0 F: 3
時間計算量
ベルマン フォード アルゴリズムの時間計算量は O(E|V| - 1) になります。
function bellmanFord(G, S) for each vertex V in G distance[V] <- 0 infinite previous[v] <- null distance[s] for each vertex v in g edge (u,v) tempdistance distance[u] + edge_weight(u, v) if < distance[v] u distance[v} error: negative cycle exists return distance[], previous[] pre> <p> <strong>Drawbacks of Bellman ford algorithm</strong> </p> <ul> <li>The bellman ford algorithm does not produce a correct answer if the sum of the edges of a cycle is negative. Let's understand this property through an example. Consider the below graph. <br> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-4.webp" alt="Bellman-Ford Algorithm"></li> <li>In the above graph, we consider vertex 1 as the source vertex and provides 0 value to it. We provide infinity value to other vertices shown as below: <br> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-5.webp" alt="Bellman-Ford Algorithm"> <br>Edges can be written as: <br> (1, 3), (1, 2), (2, 4), (3, 2), (4, 3) </li> </ul> <p> <strong>First iteration</strong> </p> <p> <strong>Consider the edge (1, 3). Denote vertex '1' as 'u' and vertex '3' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 5</p> <p>Since (0 + 5) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 5 = 5</p> <p>Therefore, the distance of vertex 3 is 5.</p> <p> <strong>Consider the edge (1, 2). Denote vertex '1' as 'u' and vertex '2' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 0</p> <p>d(v) = ∞</p> <p>c(u , v) = 4</p> <p>Since (0 + 4) is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 0 + 4 = 4</p> <p>Therefore, the distance of vertex 2 is 4.</p> <p> <strong>Consider the edge (3, 2). Denote vertex '3' as 'u' and vertex '2' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 5</p> <p>d(v) = 4</p> <p>c(u , v) = 7</p> <p>Since (5 + 7) is greater than 4, so there would be no updation in the vertex 2.</p> <p> <strong>Consider the edge (2, 4). Denote vertex '2' as 'u' and vertex '4' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 4</p> <p>d(v) = ∞</p> <p>c(u , v) = 7</p> <p>Since (4 + 7) equals to 11 which is less than ∞, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 4 + 7 = 11</p> <p>Therefore, the distance of vertex 4 is 11.</p> <p> <strong>Consider the edge (4, 3). Denote vertex '4' as 'u' and vertex '3' as 'v'. Now use the relaxing formula:</strong> </p> <p>d(u) = 11</p> <p>d(v) = 5</p> <p>c(u , v) = -15</p> <p>Since (11 - 15) equals to -4 which is less than 5, so update</p> <pre> d(v) = d(u) + c(u , v) </pre> <p>d(v) = 11 - 15 = -4</p> <p>Therefore, the distance of vertex 3 is -4.</p> <p>Now we move to the second iteration.</p> <p> <strong>Second iteration</strong> </p> <p>Now, again we will check all the edges. The first edge is (1, 3). Since (0 + 5) equals to 5 which is greater than -4 so there would be no updation in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) equals to 4 so there would be no updation in the vertex 2.</p> <p>The next edge is (3, 2). Since (-4 + 7) equals to 3 which is less than 4 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -4 + 7 = 3</p> <p>Therefore, the value at vertex 2 is 3.</p> <p>The next edge is (2, 4). Since ( 3+7) equals to 10 which is less than 11 so update</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(4) = d(2) +c(2, 4)</p> <p>= 3 + 7 = 10</p> <p>Therefore, the value at vertex 4 is 10.</p> <p>The next edge is (4, 3). Since (10 - 15) equals to -5 which is less than -4 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(3) = d(4) +c(4, 3)</p> <p>= 10 - 15 = -5</p> <p>Therefore, the value at vertex 3 is -5.</p> <p>Now we move to the third iteration.</p> <p> <strong>Third iteration</strong> </p> <p>Now again we will check all the edges. The first edge is (1, 3). Since (0 + 5) equals to 5 which is greater than -5 so there would be no updation in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) equals to 4 which is greater than 3 so there would be no updation in the vertex 2.</p> <p>The next edge is (3, 2). Since (-5 + 7) equals to 2 which is less than 3 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -5 + 7 = 2</p> <p>Therefore, the value at vertex 2 is 2.</p> <p>The next edge is (2, 4). Since (2 + 7) equals to 9 which is less than 10 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(4) = d(2) +c(2, 4)</p> <p>= 2 + 7 = 9</p> <p>Therefore, the value at vertex 4 is 9.</p> <p>The next edge is (4, 3). Since (9 - 15) equals to -6 which is less than -5 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(3) = d(4) +c(4, 3)</p> <p>= 9 - 15 = -6</p> <p>Therefore, the value at vertex 3 is -6.</p> <img src="//techcodeview.com/img/daa-tutorial/85/bellman-ford-algorithm-6.webp" alt="Bellman-Ford Algorithm"> <p>Since the graph contains 4 vertices, so according to the bellman ford algorithm, there would be only 3 iterations. If we try to perform 4<sup>th</sup> iteration on the graph, the distance of the vertices from the given vertex should not change. If the distance varies, it means that the bellman ford algorithm is not providing the correct answer.</p> <p> <strong>4<sup>th</sup> iteration</strong> </p> <p>The first edge is (1, 3). Since (0 +5) equals to 5 which is greater than -6 so there would be no change in the vertex 3.</p> <p>The next edge is (1, 2). Since (0 + 4) is greater than 2 so there would be no updation.</p> <p>The next edge is (3, 2). Since (-6 + 7) equals to 1 which is less than 3 so update:</p> <p>d(v) = d(u) + c(u, v)</p> <p>d(2) = d(3) +c(3, 2)</p> <p>= -6 + 7 = 1</p> <p>In this case, the value of the vertex is updated. So, we conclude that the bellman ford algorithm does not work when the graph contains the negative weight cycle.</p> <p>Therefore, the value at vertex 2 is 1.</p> <hr></->
d(v) = 0 + 5 = 5
したがって、頂点 3 の距離は 5 になります。
エッジ (1, 2) を考えてみましょう。頂点 '1' を 'u' として、頂点 '2' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 0
d(v) = ∞
c(u , v) = 4
(0 + 4) は ∞ 未満なので、更新します
d(v) = d(u) + c(u , v)
d(v) = 0 + 4 = 4
したがって、頂点 2 の距離は 4 になります。
エッジ (3, 2) を考えてみましょう。頂点 '3' を 'u' として、頂点 '2' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 5
d(v) = 4
c(u , v) = 7
(5 + 7) は 4 より大きいため、頂点 2 には更新はありません。
エッジ (2, 4) を考えてみましょう。頂点 '2' を 'u' として、頂点 '4' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 4
d(v) = ∞
c(u , v) = 7
(4 + 7) は 11 に等しいので、∞ より小さいため、更新します。
d(v) = d(u) + c(u , v)
d(v) = 4 + 7 = 11
したがって、頂点 4 の距離は 11 になります。
エッジ (4, 3) を考えてみましょう。頂点 '4' を 'u' として、頂点 '3' を 'v' として表します。ここでリラックス公式を使用します。
d(u) = 11
d(v) = 5
c(u , v) = -15
(11 - 15) は -4 に等しいため、5 より小さいため、更新します。
d(v) = d(u) + c(u , v)
d(v) = 11 - 15 = -4
したがって、頂点 3 の距離は -4 になります。
次に、2 番目の反復に進みます。
2 回目の反復
ここで、もう一度すべてのエッジを確認します。最初のエッジは (1, 3) です。 (0 + 5) は -4 より大きい 5 に等しいため、頂点 3 には更新はありません。
次のエッジは (1, 2) です。 (0 + 4) は 4 に等しいため、頂点 2 には更新はありません。
次のエッジは (3, 2) です。 (-4 + 7) は 3 に等しいため、4 より小さいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(2) = d(3) +c(3, 2)
= -4 + 7 = 3
abcと数字
したがって、頂点 2 の値は 3 になります。
次のエッジは (2, 4) です。 ( 3+7) は 10 に等しいため、11 より小さいため、更新します
d(v) = d(u) + c(u, v)
d(4) = d(2) +c(2, 4)
= 3 + 7 = 10
したがって、頂点 4 の値は 10 になります。
部分文字列 java
次のエッジは (4, 3) です。 (10 - 15) は -4 より小さい -5 に等しいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(3) = d(4) +c(4, 3)
= 10 - 15 = -5
したがって、頂点 3 の値は -5 になります。
次に、3 回目の反復に進みます。
3回目の反復
ここでもう一度すべてのエッジを確認します。最初のエッジは (1, 3) です。 (0 + 5) は -5 より大きい 5 に等しいため、頂点 3 は更新されません。
次のエッジは (1, 2) です。 (0 + 4) は 3 より大きい 4 に等しいため、頂点 2 は更新されません。
次のエッジは (3, 2) です。 (-5 + 7) は 2 に等しいため、3 より小さいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(2) = d(3) +c(3, 2)
= -5 + 7 = 2
したがって、頂点 2 の値は 2 になります。
次のエッジは (2, 4) です。 (2 + 7) は 9 に等しいため、10 より小さいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(4) = d(2) +c(2, 4)
= 2 + 7 = 9
したがって、頂点 4 の値は 9 になります。
次のエッジは (4, 3) です。 (9 - 15) は -5 より小さい -6 に等しいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(3) = d(4) +c(4, 3)
= 9 - 15 = -6
したがって、頂点 3 の値は -6 になります。

グラフには 4 つの頂点が含まれているため、ベルマン フォード アルゴリズムによれば、反復は 3 回のみとなります。 4を実行しようとすると番目グラフ上の反復では、指定された頂点からの頂点の距離は変化しないはずです。距離が異なる場合は、ベルマン フォード アルゴリズムが正しい答えを提供していないことを意味します。
4番目反復
最初のエッジは (1, 3) です。 (0 +5) は -6 より大きい 5 に等しいため、頂点 3 には変化はありません。
次のエッジは (1, 2) です。 (0 + 4) は 2 より大きいため、更新は行われません。
次のエッジは (3, 2) です。 (-6 + 7) は 1 に等しいため、3 より小さいため、次のように更新します。
d(v) = d(u) + c(u, v)
d(2) = d(3) +c(3, 2)
= -6 + 7 = 1
この場合、頂点の値が更新されます。したがって、グラフに負の重みサイクルが含まれる場合、ベルマン フォード アルゴリズムは機能しないと結論付けます。
したがって、頂点 2 の値は 1 になります。
